Scale factor is an important index of MEMS gyroscope, which means that in the range of MEMS gyroscope input angular velocity, the output of the gyroscope relative to the maximum deviation of the least square fitting line and the maximum output in the range of the MEMS gyroscope. This paper will analyze the nonlinear degree of open loop scale factor and closed loop scale factor, and will briefly analyze its flow, formula and meaning.
Open-loop scale factor nonlinear degree analysis
The gyroscope is in the open-loop detection mode, and its detection principle diagram is shown in Figure 1.
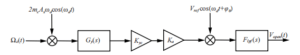
Figure 1. Gyroscope open-loop detection model
Its static sensitivity is:
![]()
In the formula, Δωis the frequency difference between gyro modes, Ax is the drive displacement amplitude, Kyc is the detection mode displacement to capacitance conversion gain, Ka = KcvKamp is the detection loop capacitance-voltage conversion gain and front-end amplification gain, Vref is the amplitude of phase-sensitive demodulation reference, and F1pf(0) is the low-pass filter gain.
For the nonlinearity of the scale factor of the gyroscope under normal temperature studied in this paper, the effect of temperature on it is ignored. The essence is that the static sensitivity of the gyroscope will change correspondingly when the input angular velocityΩof the Z-axis is different, which is manifested as the nonlinearity of the scale factor in the range of the gyroscope. Then take this as the premise to analyze the influencing factors in the above formula one by one:
(1) For Δω. In the dynamic equation of vibratory gyroscope, there is a term that the input angular velocity affects the equivalent stiffness of the driving mode and the detection mode, which will affect the driving frequency and detection frequency, and then affect the difference between modes Δω.
(2) For Ax. When the gyroscope is working normally, because the drive loop uses AGC control loop to control the drive amplitude of the gyroscope, it can be considered that the drive amplitude does not change with the input angular velocity.
(3) For Kyc. The nonlinear term here may cause the scale factor of the gyroscope to be nonlinear.
(4) For Ka. The nonlinear term is also simplified in the correlation derivation of the annular diode circuit used in the capacitor-voltage conversion process, where the nonlinear scaling factor may also be caused.
For Vref and F1pf(0). Because the expressions of these two terms do not have terms related to the input angular velocity, it can be considered that their values do not change with the input angular velocity.
Closed-loop scale factor nonlinear degree analysis
When the gyroscope detection mode works in closed loop mode, the static sensitivity is shown as the following formula:
![]()
In the formula, mc is the size of the gyroscope's Coriolis mass, Ax is the driving displacement amplitude, ωd is the driving frequency, and Kvf is the voltage to electrostatic force conversion link gain.
It can be seen from the above formula that, thanks to the advantages of closed-loop control, compared with open-loop detection, the closed-loop scaling factor of gyroscope will not be affected by the gain Kyc and Kcv of the two links with the greatest influence on nonlinearity analyzed in the previous section, which also reflects the advantages of the closed-loop system in reliability and stability.
The following will use the method of analyzing the open-loop detection of nonlinearity, according to whether the input angular velocity of the gyroscope will have an impact on the parameters in the above formula, the parameters will be briefly analyzed one by one:
(1) For mc. For a given gyroscope, its mass generally does not change with the angular velocity.
(2) For Ax. When the gyroscope is working normally, because the drive loop uses AGC control loop to control the drive amplitude of the gyroscope, it can be considered that the drive amplitude does not change with the input angular velocity.
(3) For ωd. When the gyroscope is working normally, under the closed-loop control of the drive loop, the drive mode will always be in a resonant state, and there is ωd=ωx.
(4) For Kvf. The voltage-electrostatic conversion part discussed here includes the generating circuit of the force feedback voltage and the detecting force feedback comb, both of which may introduce nonlinearities related to the input angular velocity.
Conclusion
Scale factor, one of the important indexes of MEMS gyroscope, is introduced in this paper. The key indexes of MEMS gyroscope are analyzed from the perspective of open loop scale factor nonlinearity and closed loop scale factor nonlinearity. The scale factor repeatability of ER-MG2-50/100 is <200ppm, and the scale factor repeatability of ER-MG2-300/400 is <100-300 PPM. The excellent performance of MEMS gyroscope provides adequate technical support for ground, air and sea navigation.
I hope that through this article you can only understand the MEMS gyroscope, if you want to learn more, please click the related articles and products below.
More Technical Questions
1.Analysis of drive loop noise of MEMS gyroscope
2.Basic dynamics analysis of MEMS gyroscope
3.Bias Temperature Compensation Analysis of MEMS Gyroscope
4.System error and calibration of MEMS gyroscope
5.Sensitive structure analysis of MEMS gyroscope
6.The impact of turntable error on MEMS gyroscope calibration
Products in Article







